Simcenter Testing Solutions Sound Quality Metrics: Loudness and Sones

Direct YouTube link: https://youtu.be/zy7z94nHknA


Sones is unit of measure of the loudness of a sound. Sones is a different unit of measure than the traditional decibel quantity. 

Sones takes into account the frequency and level dependent nature of human hearing, while decibels does not fully address this dependency.

Contents:
1. How Loud is a Sound?
2. The Human Hearing Domain
3. Loudness as a Sound Metric
4. The Loudness Unit: Phons and Sones
5. Practical Example: Vacuums
6.  Loudness Representations
   6.1 Single Number
   6.2 Time Varying Loudness
   6.3 Stationary Loudness Spectrum (Specific Loudness)
   6.4 Loudness Map
7. Loudness versus A-Weighting
8. Loudness Standards
9. Calculating Loudness in Simcenter Testlab
   9.1 Calculating Loudness in a Display
   9.2 Loudness in Simcenter Testlab Neo
   9.3 Calculating Loudness in Signature Throughput Processing
10. Conclusion


1. How Loud is a Sound?

The decibel value is often used to quantify sound. Decibel (dB) values accurately represent the amplitude of a sound, but they do not accurately represent the perceived loudness of a sound.

In fact, there is a separate sound quality metric called loudness (with units sones or phons) which gives a much better representation of how humans perceive the level of a sound.

2. The Human Hearing Domain

The human hearing domain is shown in Figure 1.

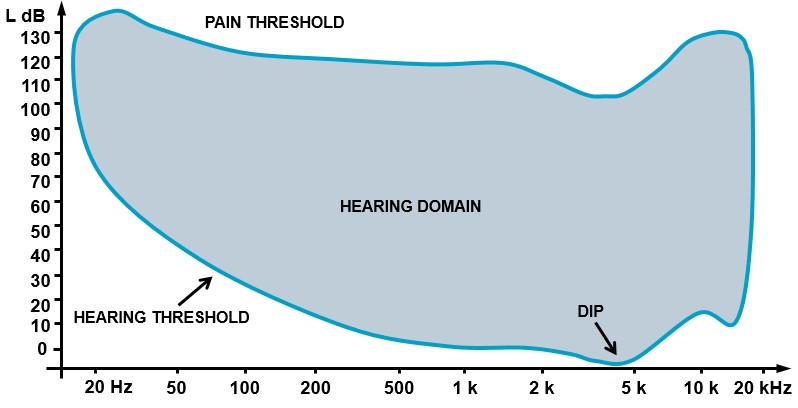
Figure 1: The human hearing domain is both frequency and level dependent. Sounds cannot be heard at the decibel levels and frequencies outside of the grey area. The dip at about 4000 Hz is because the air volume in the ear canal goes into resonance which allows the ear to hear even low level sounds.

Tracing the lower limit (the hearing threshold), it is evident that the lower limit of hearing varies with frequency. The threshold has different values at different frequencies.

There is a "dip" between 3000Hz-5000Hz. Humans can hear particularly well at these frequencies due to a resonance of the air volume in the ear canal. In general, humans can hear sounds at lower decibel levels between 3 kHz and 5 kHz than at any other frequency.

For example, humans are able to hear a 10dB sound at 5 kHz but we cannot hear a 10dB sound at 50Hz as shown in Figure 2.

Figure 2: A tone at 50Hz and 10dB is inaudible. A tone at 5000Hz and 10dB is audible.

The dB value is the same at both frequencies, but the perceived loudness is very different – one is audible, the other is inaudible. Clearly dB alone is insufficient to represent the perceived loudness of a sound.

3. Loudness as a Sound Metric

The loudness metric is based off of perceived loudness. Thus the metric was developed with a jury of humans (unlike decibels which is simply a math equation).

Each curve shown on Figure 3 below represents a curve of equal loudness for sinusoidal tones. As frequency changes along a curve, the dB value also must change to result in an equally loud sound.

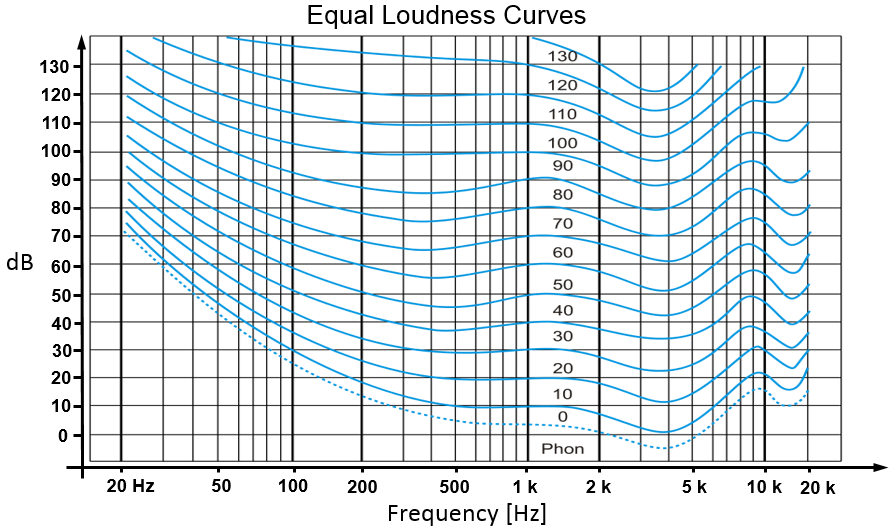
Figure 3: The curves of equal loudness originally developed by Fletcher-Munson in 1933.

To develop this metric, a jury of humans with normal hearing was gathered. The jury would listen to a tone at 1000Hz and a particular dB level. Then, a second tone would be played at a different frequency. The level of the second tone would be altered until it sounded equally as loud as the 1000Hz tone.

For example, a 1000Hz tone was played at 40dB. Then a 100Hz tone was played and the volume was adjusted until it sounded equally as loud to the jury as the 1000Hz tone. The 100Hz tone would have to be playing at 52dB to sound equally as loud as the 1000Hz tone at 40dB (see Figure 4).

Figure 4: To develop the curves, two tones were played and the levels adjusted until they sounded equally as loud.

This jury experiment was repeated until all frequencies and 13 decibel ranges within the human hearing domain were included. This resulted in several curves of equal loudness.

A curve of equal loudness represents all the frequencies and dB levels that are perceived as equally as loud. In Figure 5 below, every frequency and dB level that lies on the pink curve is perceived as equally as loud.

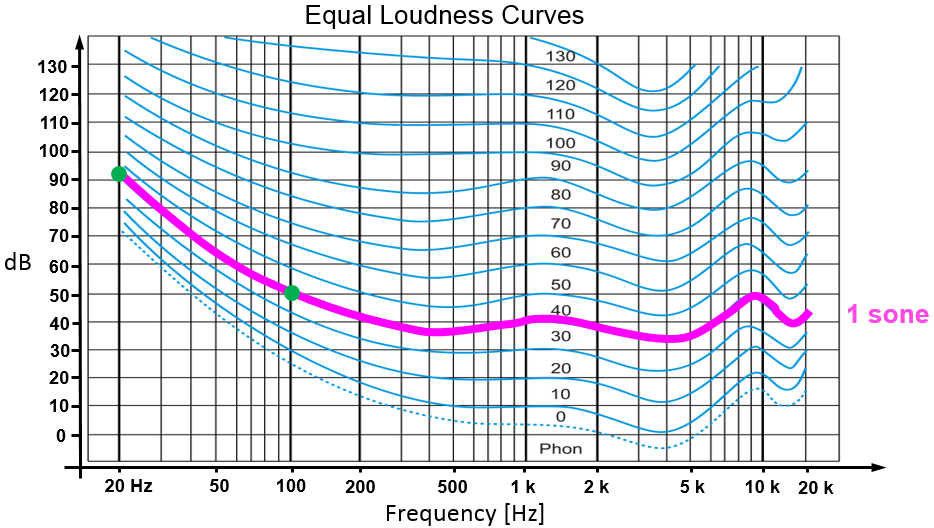
Figure 5: The curve of equal loudness represent dB and frequency values that are perceived as equally loud (Magenta is a 1 sone curve of equal loudness).

This means that a 100 Hz tone at 50 dB sounds equally as loud as a 20 Hz tone at 92 dB as they both intersect the pink curve at these respective frequencies and decibels levels. Both the 50 dB tone (100 Hz) and 92 dB tone (20 Hz) can be expressed as 1 sone or 40 phons.

At 1000Hz, the curves of equal loudness are 10dB, or 10 phons, apart. This means that at 1000Hz, an increase of 10dB corresponds to a doubling in perceived loudness.

The “rule of thumb” that a 10dB increase corresponds to a doubling in perceived loudness does not hold true at all frequencies. At 30Hz a doubling in perceived loudness only requires a 5dB increase. However, a doubling of sone level does correspond to a doubling of perceived loudness at all frequencies. This can be seen in Figure 6.

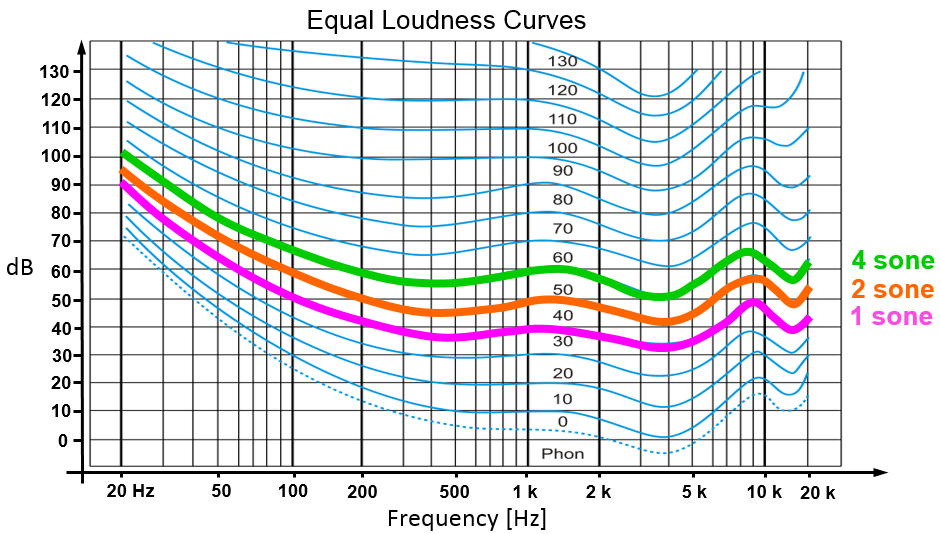
Figure 6: The sone scale is frequency independent. A doubling of sones equates to a doubling of perceived loudness at any frequency.

The sone scale is linear, so no matter the frequency or level, 2 sones is twice as loud as 1 sone, and 4 sones is twice as loud as 2 sones. This doubling is more consistent than the decibel scale where it is only a "general rule of thumb" that 10 dB is doubling in perceived sound.

4. The Loudness Unit: Phons and Sones

Loudness level can be expressed in sones or phons, which are both units of loudness.

The phon is a unit of loudness that represents equal loudness to a 1000Hz tone. This means that if a tone is 90 phons it is equally loud as a 1000Hz tone at 90dB. If a 125 Hz tone is 47 phons, it is equally as loud as a 1000Hz tone at 47dB.

The sone is the other unit of equal loudness. The conversion between phons and sones is shown in Equation 1:

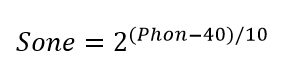
Equation 1: Relationship between phons and sones.

The sone is typically preferred over the phon because it is a linear unit. This means that if the sone value triples, the perceived loudness triples. This holds true across the entire frequency range.

Phons do not scale linearly with perceived loudness. An increase of 10 phons represents a doubling in perceived loudness.

The sone scale is considered more intuitive.

• The difference between 60 and 70 phones is not a logical doubling in perceived loudness.
• The difference between 4 sones and 8 sones is a logical doubling in perceived loudness.

5. Practical Example: Vacuums

To demonstrate the value of sones versus decibels, listen to the video below. It is recommended to listen with high quality headphones rather than laptop speakers.

Sounds from two different vacuums will play. The playback order is as follows:

• Vacuum A: 4 seconds
• Vacuum B: 4 seconds
• Vacuum A: 4 seconds
• Vacuum B: 4 seconds

Listen to the recording and decide for yourself which vacuum sounds louder.


Direct YouTube link: https://youtu.be/1pI9wFbMmfQ

 

Many listeners remark that vacuum brand A sounds louder than vacuum brand B. Many say this is due to the “loud” tone in vacuum brand A shown in Figure 7.

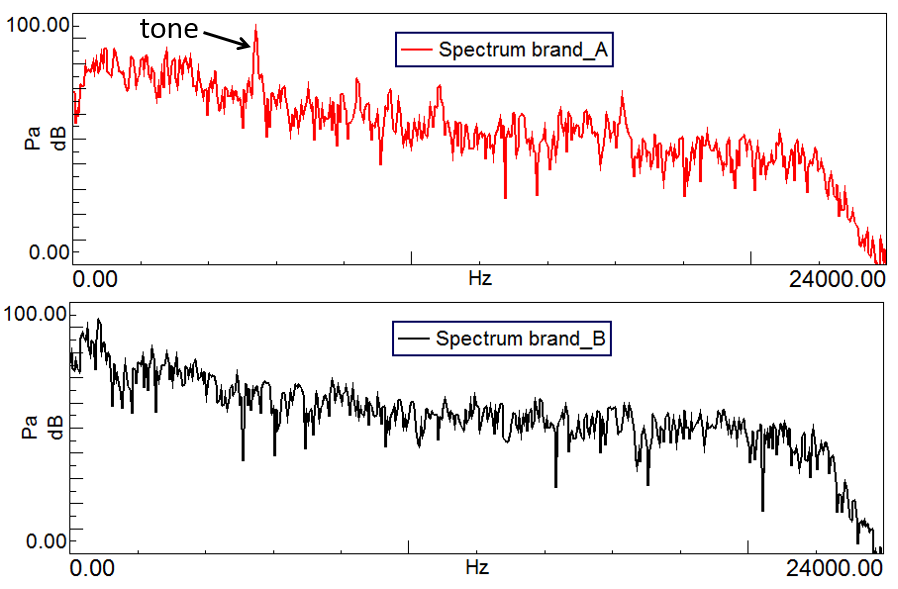
Figure 7: Spectrums of vacuum brand A and brand B. Brand A has a “loud” tone around 5500Hz.

 

Figure 8 below shows the results of analyzing both Vacuum A and Vacuum B.  Results in both decibels and sones were calculated.

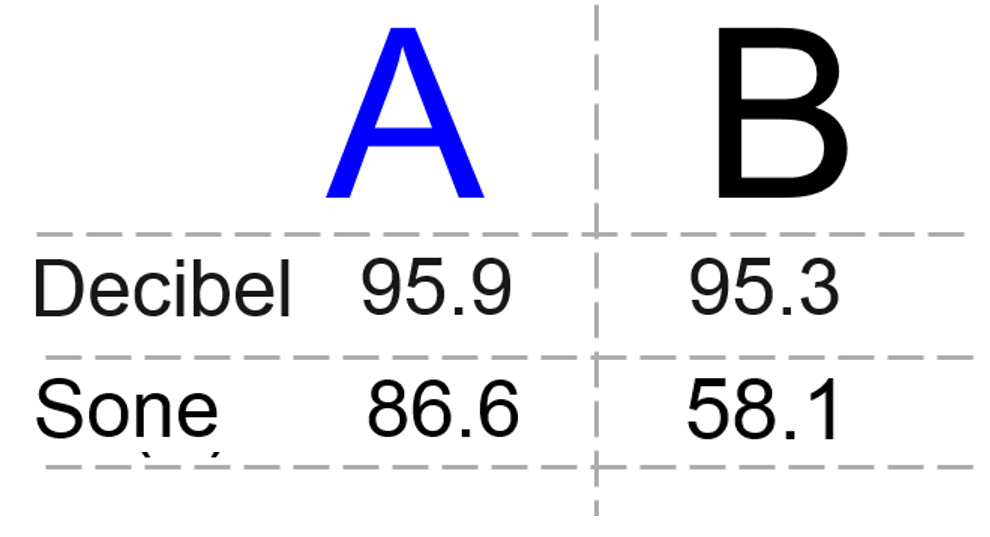
Figure 8: Comparison of decibel and sone results from vacuum comparison.

The results indicate:

• Vacuum brand A and brand B have virtually the same decibel level.
• Vacuum brand A has a higher sone level than vacuum brand B.

In fact, the sone level calculated considers vacuum B to be perceived as ~30% quieter than vacuum brand A! The sone calculation more closely matches human perception of the sound.

6. Loudness Representations

Loudness results from the analysis of a sound signal can be formatted and shown several different ways:

6.1 Single Number

A single value for loudness, express in sones, can be derived from a sound spectrum as shown in Figure 9.

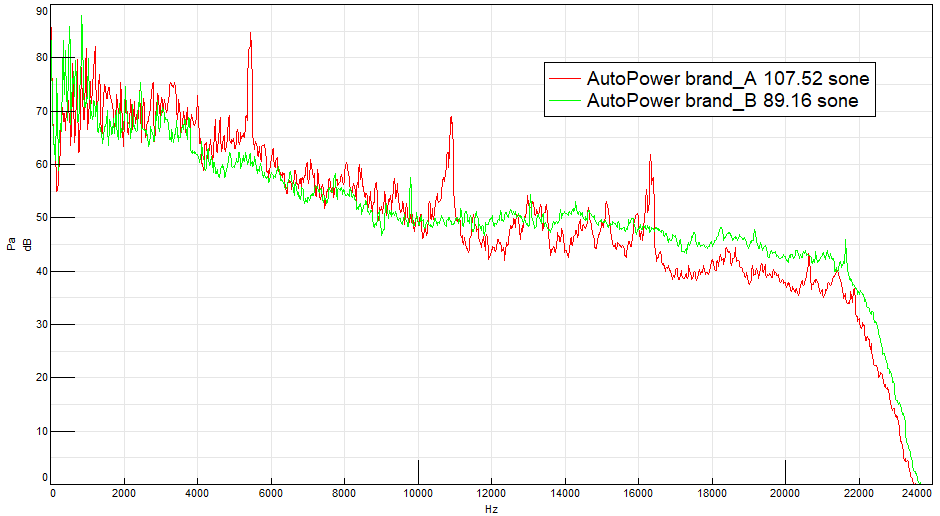
Figure 9: Legend (upper right) shows a single loudness value in sones which represents the total loudness for the sound spectrum.

Distilling the loudness value to a single number can be useful for comparing signals quickly at a high level.

6.2 Time Varying Loudness

The loudness of sound signal can be calculated over time.  This is known as Time Varying Loudness (Figure 10).

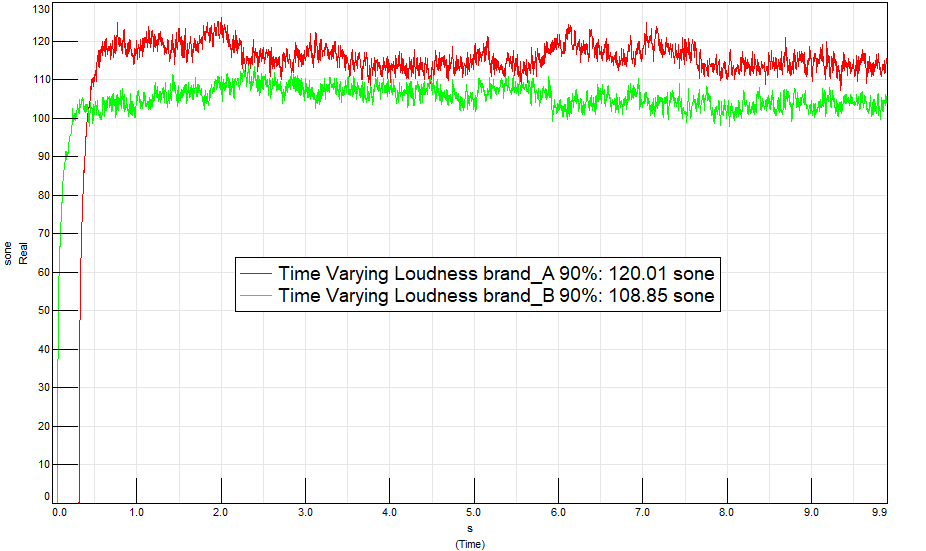
Figure 10: Time varying loudness results.

It is useful to analyze transient signals with time varying loudness.  Often, the 90% percentile of the loudness values over time is calculated.  This is called the N10 loudness (N is short for loudness, 10 is 90th percentile where 10% of all loudness values over time are above the N10 number).

Some time domain event can be covered over by temporal masking effects of human hearing.  More information here: Auditory Masking.

6.3 Stationary Loudness Spectrum (Specific Loudness)

The loudness level of the twenty four different critical bands of human hearing can also be used to diagnosis a sound signal (Figure 11).
 

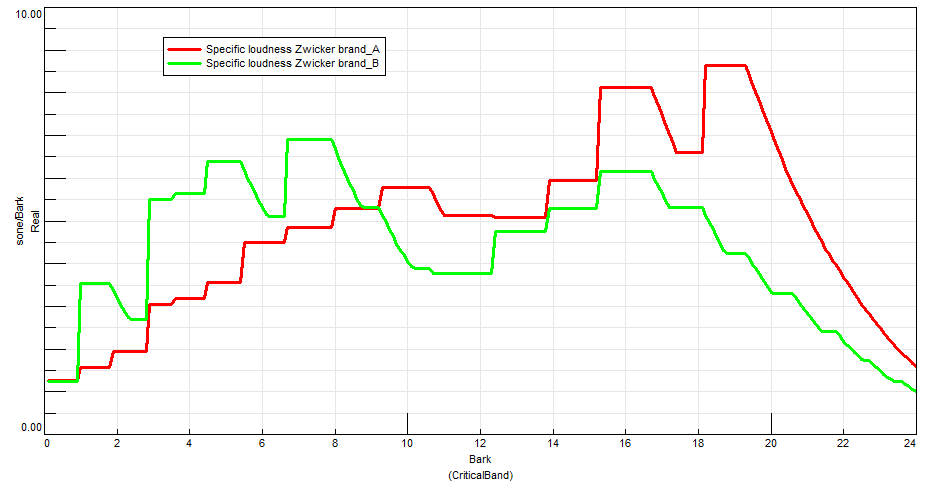
Figure 11: Specific Loudness spectrums.  Sones on Y-axis, critical bark bands on X-axis.

In this case, the sound of Brand A (red curve) is much louder at high frequencies than the sound of Brand B (green curve). Vice versa is also true.  Brand B (green) has louder frequency content at low frequencies than Brand A (red curve).

The stationary loudness spectrum is useful to located the frequency ranges that contribute the most to the perceived loudness of a sound.  This spectrum is often called a "specific loudness spectrum".

More about human hearing critical bands: Critical Bands in Human Hearing.

6.4 Loudness Map

Instead of a single stationary loudness spectrum, loudness per critical band can also be calculated over time over rpm as shown in Figure 12.
 

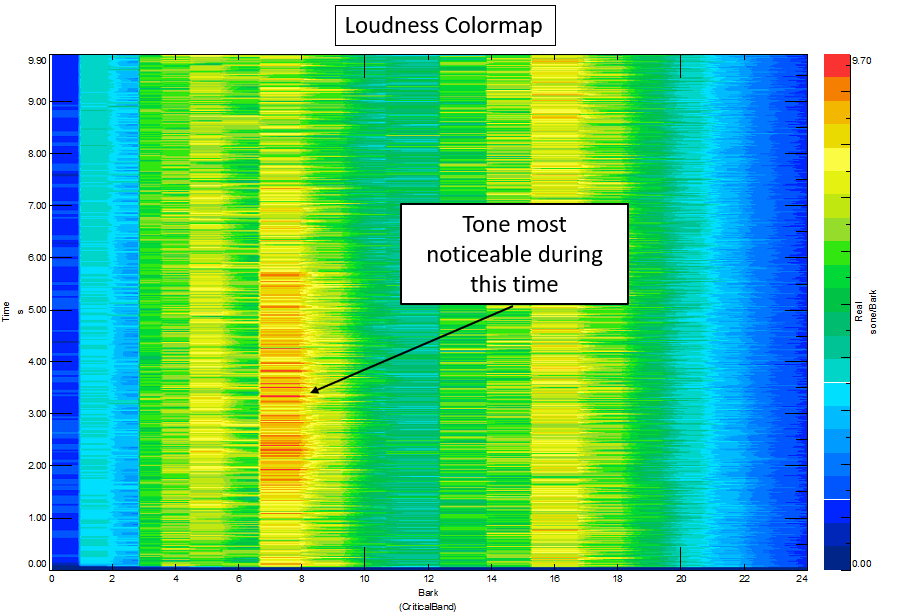
Figure 12: Loudness per critical band over time (or can be versus rpm).

This can help locate both the time and the frequency range where the largest contributor to loudness occurs.

7. Loudness versus A-Weighting

It would be unfair to talk about loudness and decibels without mentioning the A-weighted decibel scale.

In an attempt to make decibels more closely match human perception of loudness, the A-weighted decibel scale was developed (Figure 13). Essentially, the A-weighting curve adjusts the dB level at different frequencies to make it more closely match the perceived loudness.

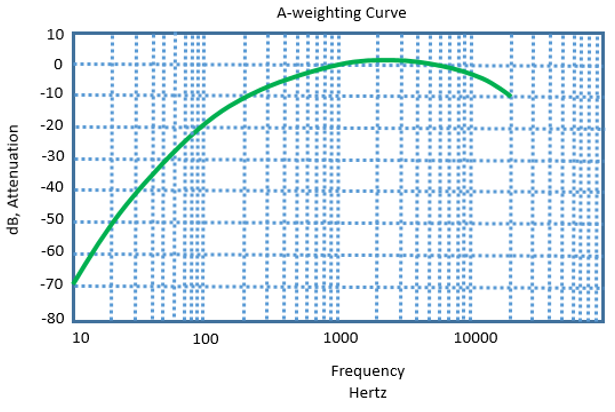
Figure 13: A-weighting curve (green) consists of dB of attenuation versus frequency.

Depending on the frequency, the dB level is either attenuated or accentuated. This new dB value, the A-weighted dB value, is supposed to more closely match the perceived loudness.

Imagine measuring a 50 Hz tone at two different frequencies (Figure 14). According to the A-weighting scale below, the measured dB value should be subtracted by 30dB to more closely match human perception of the sound (red dot). Alternatively, imagine measuring a 5000 Hz tone. According to the A-weighting scale, the measured value does not need to be adjusted (green dot).

Figure 14: The A-weighting curve (green) attenuates or accentuates dB measurements based on frequency. Red dot is 30 dB of attenuation. Green dot is 0 dB of attenuation.

If the A-weighted curve is compared to the human hearing threshold (Figure 15), it attempts to follow the shape of the threshold. However, due to limitation of creating filters in analog circuits, the curve had to be simplified.

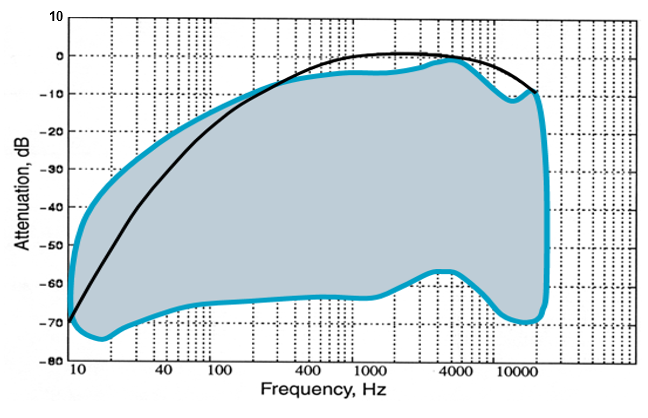
Figure 15: The A-weighting curve (black) is much simpler than the equal loudness curves (outlined in blue with grey, inverted).

There is no such simplification in the loudness curves, unlike the A-weighting curve.

Furthermore, the A-weighting curve does not change as a function of sound level, like the equal loudness curves shown previously in the article.

The A-weighting decibel value is more representative of perceived loudness than linear decibels. However the loudness metric is still the superior qualifier of perceived loudness due to the more detailed adjustment of the equal loudness curves.

If we added the A-weighted decibel value to the vacuum results chart from above it would appear as follows (Figure 16):

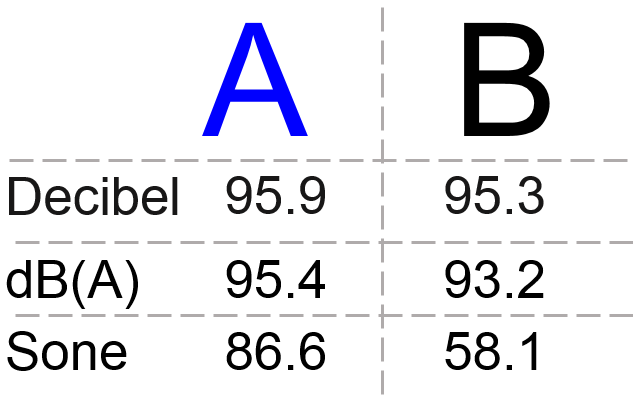
Figure 16: A results comparison using dB, dB(A), and sones.

The A-weighted decibels showed more of a difference than the linear decibels, but still not as much of a difference as sones.

More about A-weighting in this article: What is A-weighting?.

8. Loudness Standards

There are different standards for the calculation of sones that can be used:

• Stevens Mark VI and VII – Professor Stanley Smith Stevens of Harvard published his Mark VI and Mark VII standards for sone calculations in 1961 and 1975 respectively.
• ISO532B – Standard was first published in 1975 and incorporated masking calculations proposed by Professor Eberhard Zwicker.
• DIN 45631 – The German standards organization, Deutsches Institut für Normung (DIN), also maintains a commonly referenced standard for the calculation of loudness.

The ISO532B and DIN 45631 standard include considerations for auditory masking.  The loudness total does not include any content of the sound that does not contribute to the total loudness.  More about masking in this knowledge article: Auditory Masking.

These methods outlined in these standards can produce different results for loudness. All methods for calculating loudness are available in Simcenter Testlab.

9. Calculating Loudness in Simcenter Testlab

There are several option to calculate loudness in Simcenter Testlab (previously called LMS Test.Lab):

9.1 Calculating Loudness in a Display

In a display with a sound spectrum plotted, right click on the legend and select “Options” as shown in Figure 17.
 

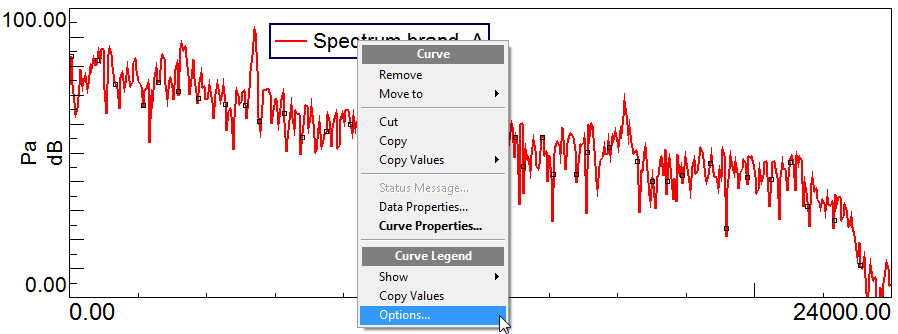
Figure 17: Select “Options…” by right clicking in display.

In the "Curve Legend Options" dialog that appears, choose the “Calculated Content" tab as shown in Figure 18. 
 

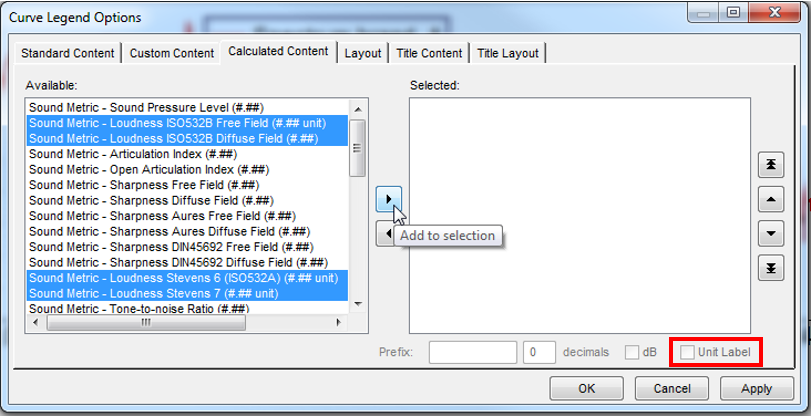
Figure 18: Choose the metric to be calculated from the list on the left and use the arrows to move them to the visible list on right.

Select the type of loudness calculation to be performed from the list.

Available loudness calculations include ISO532B Free Field, ISO 532B Diffuse Field, Stevens 6, and Stevens 7. Click the “Add to Selection” arrow to calculate the metric. Check on the “Unit Label” box to display the unit in the legend.

Press OK when finished. The sone value for the sound spectrum will be displayed as shown in Figure 19.
 

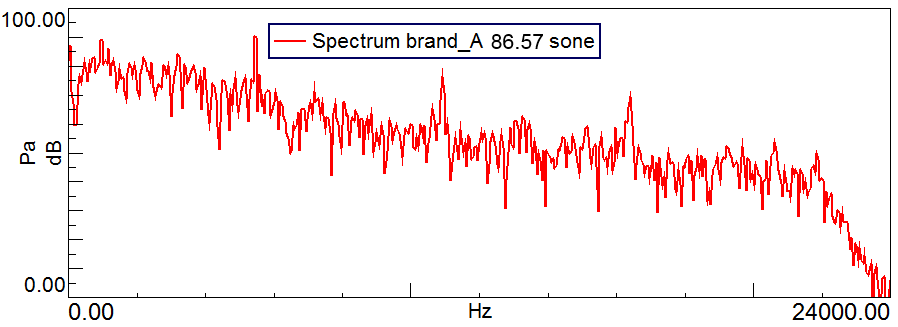
Figure 19: The value and unit will be displayed in the legend.

This functionality also works in a active picture report.  More about active pictures in the knowledge article: Simcenter Testlab Active Picture.

9.2 Loudness in Simcenter Testlab Neo


Direct YouTube link: https://youtu.be/pjcNH3oa560

Simcenter Testlab Neo has several methods for calculating loudness as shown in Figure 20.

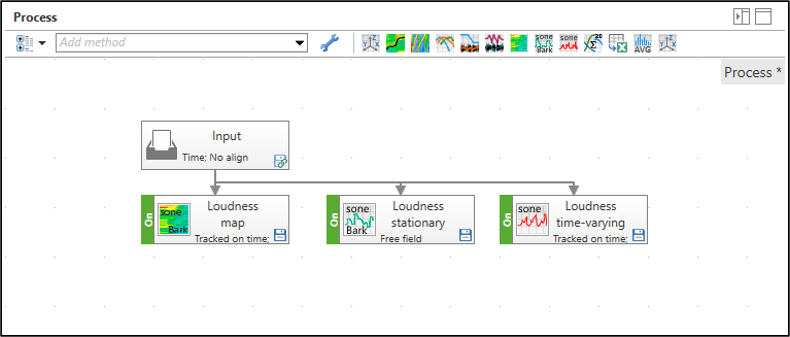
Figure 20: Simcenter Testlab Neo methods for loudness.

Methods include stationary loudness spectrum, loudness map, and time varying loudness.

More about creating processed and analyzing data using SImcenter Testlab Neo in these knowledge articles:

• Simcenter Testlab Neo Introduction
• Simcenter Testlab Neo: Process Designer

9.3 Calculating Loudness in Signature Throughput Processing

To calculate loudness in Signature Throughput Processing, turn on three add-ins: ANSI-IEC Octave filtering, Signature Throughput Processing, and Sound Quality Metrics as shown in Figure 21.
 

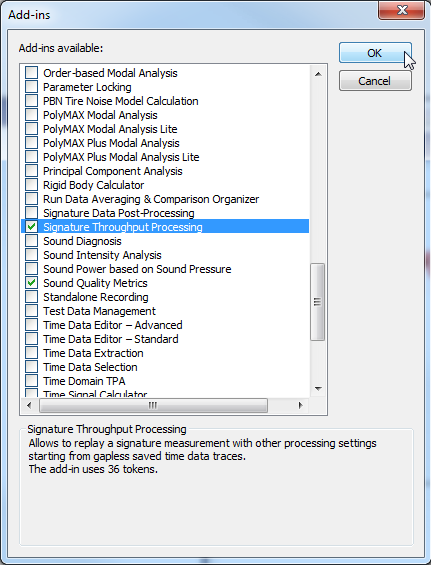
Figure 21: ANSI-IEC Octave filtering requires 23 tokens. Sound Quality Metrics requires 33 tokens. Signature Throughput Processing requires 36 tokens.

In Simcenter Testlab Time Data Processing worksheet, under “Section Settings”, turn on the loudness metrics to be calculated (Figure 22).

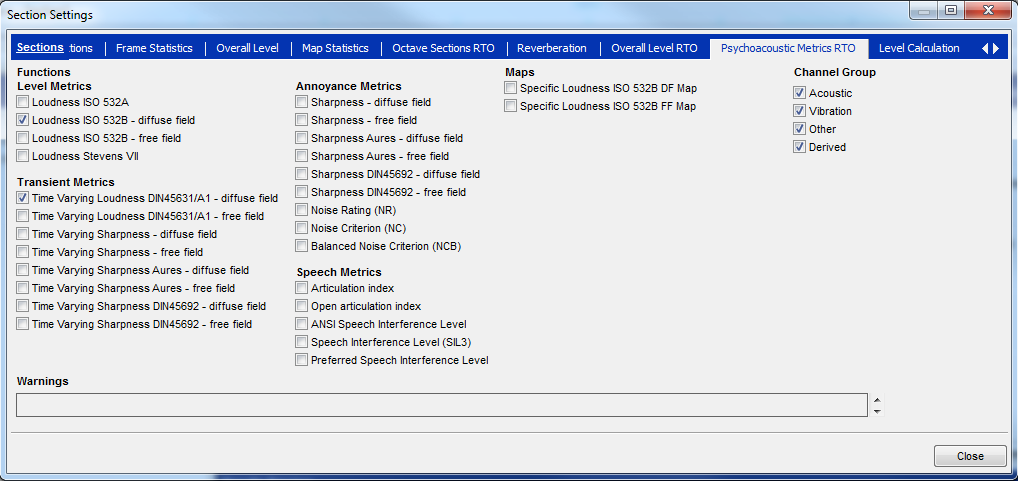
Figure 22: Throughput processing -> Section Settings -> Psychoacoustic Metrics

Note that time varying loudness according to DIN 45631, the most popular loudness formulation, is only available in Throughput Processing in Simcenter Testlab classic.

More information about processing Time Varying Loudness according to the DIN standard, and calculating a N10 value from it, see the Forum post: How do I calculate N10 Time Varying Loudness in Simcenter Testlab?.

Time Varying Loudness also accounts for temporal masking.  More information in the knowledge article: Masking.

A specific loudness spectrum can also be calculated. See the Forum post: How to Calculate a Specific Loudness spectrum in Simcenter Testlab?

10. Conclusion

The loudness metric, expressed in units of sones, is a great way to more closely match people’s perception of hearing. Decibels, and even A-weighted decibels, do not adjust enough to fully represent the human hearing dependency on level and frequency to give a representative value of the perceived loudness.

 

Questions? Email Scott MacDonald (macdonald@siemens.com), post a reply, or contact Siemens Support Center.

 

Related Links:

• Index of Testing Knowledge Articles
• History of Acoustics
• Sound Pressure
• What is a decibel?
• What is A-weighting?
• Octaves and Human Hearing
• Auditory Masking
• Articulation Index
• Tonality
• Tone-to-Noise Ratio and Prominence Ratio
• Fluctuation Strength and Roughness
• Sharpness
• Simcenter Testlab Neo: Modulation Metrics
• Critical Bands in Human Hearing
• How to Calculate N10 Time Varying Loudness in Simcenter Testlab?
• Calculating a Specific Loudness Spectrum in Simcenter Testlab
• Kurtosis
• Sound Quality Jury Testing
• Alter Speed of Rotating Sound for Listening
• What is Sound Power?
• Decibel Math
• What is the Acoustic Quantity called Q?
• Sound Absorption
• Sound Transmission Loss
• Noise level certification, how to select the right standard?
• Sound pressure, sound power, and sound intensity: What is the difference?
• Sound fields: Free and diffuse field, near and far field
• Describing Sounds with Words
• Simcenter Testlab Pass-by Noise Testing
• Sound Quality YouTube Playlist
• Sound Metrics Unleashed Seminar
• Sound Quality YouTube Playlist